After the post on Assessing Bar Model Solutions went up, Beth Curran sent a message: “We just did that problem!” She agreed to share some student work:
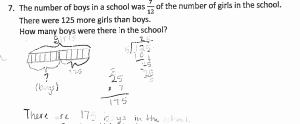
And when the students didn’t draw a model:
I see this as a comparison problem:
5 units -> 125 students
1 unit -> 125 ÷ 5 = 25
7 units for boys -> 7 x 25 = 175 boys in all
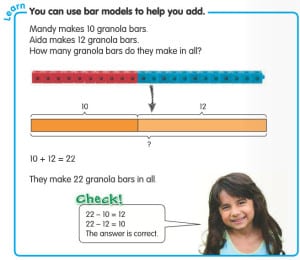
(That’s the Thinking Blocks Model Drawing tool that allows you to insert your own word problems and solve – or you can use the pre-made questions!)

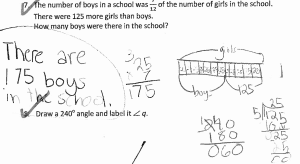
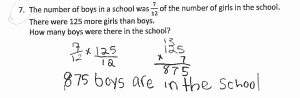



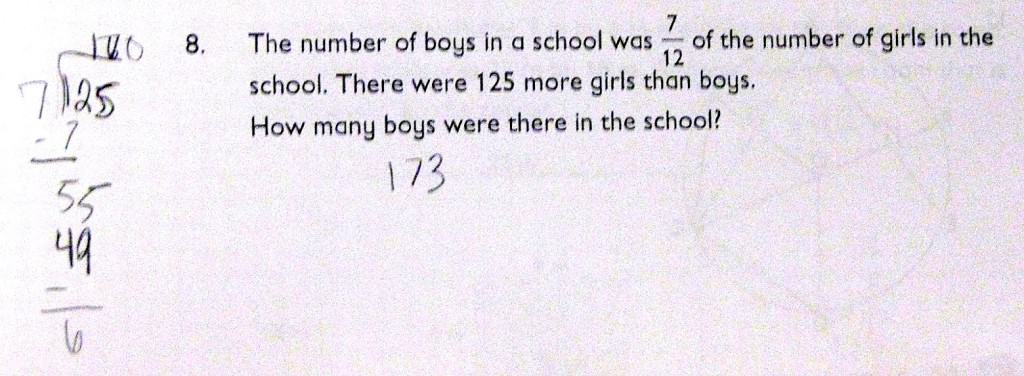

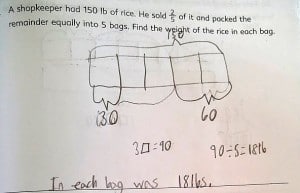



 For 2015, I submitted similar proposals to both NCTM and NCTM…and BOTH were accepted. Woot! And good news, my co presenter will be Lauri Susi of
For 2015, I submitted similar proposals to both NCTM and NCTM…and BOTH were accepted. Woot! And good news, my co presenter will be Lauri Susi of 
 2015 NCSM Annual Conference – April 13-15, 2015
2015 NCSM Annual Conference – April 13-15, 2015