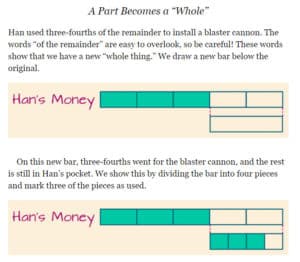
Over the summer, we thought it would be fun to run some of the most popular posts from the past. When I re-read a post from the past I always take away something different because I am in a different place with my own experience. Perhaps you are as well!
Personal Whiteboards
Originally published 3/25/2009
In the post about Number Strings, I referred to a student’s “personal whiteboard”. I use whiteboards throughout the day as a way of informally assessing students.
Instead of a store bought whiteboard, I prefer to provide students with a customized version.
- Start with a glossy page protector, a box of which can be purchased inexpensively on eBay or at Sam’s Club or Costco.
- Insert a brightly colored sheet of card stock. Cardstock makes the whiteboard a little sturdier and by using color on one side, I can instantly tell when the entire group of students is ready.
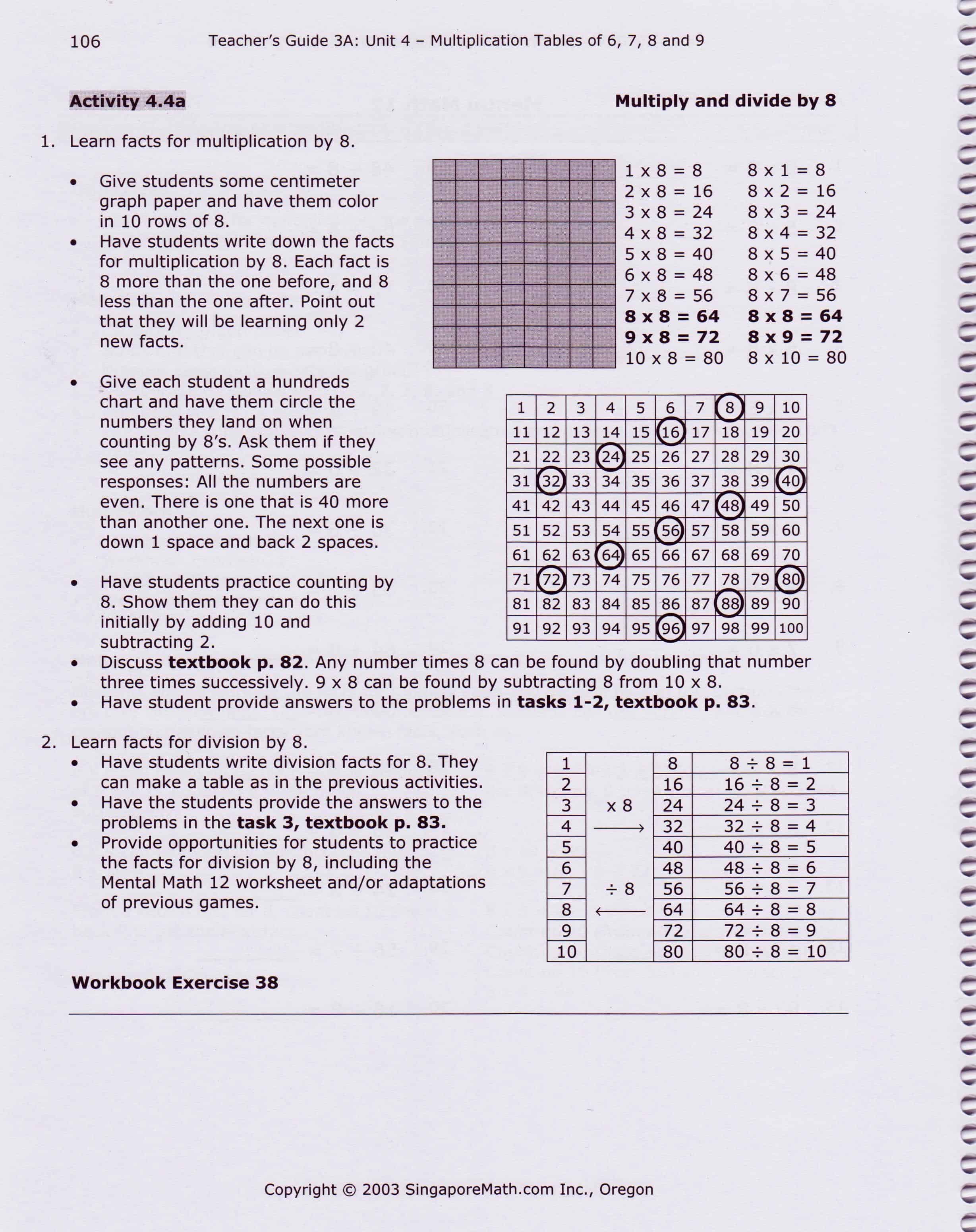
- Add appropriate pages. In the first grade, I might have a pre-made number bond page ready to go. When I’m teaching a lesson on adding or subtracting, I’ll insert a place value chart.
By keeping a classroom set of these on the shelf with the student textbooks, they would last an entire school year. Here are some printables to get you started:
- Number Bond
- Ones/Tens Place Value Chart
- Ones/Tens/Hundreds Place Value Chart
- Isometric dot paper
- 1 or 4 Quadrant Graph Paper
You can find information on Alexandria Jones’ Pharaoh’s Treasure in the picture at Let’s Play Math.
These are also great for games and learning centers…
Or any of the international logic games on the handouts page of this site.











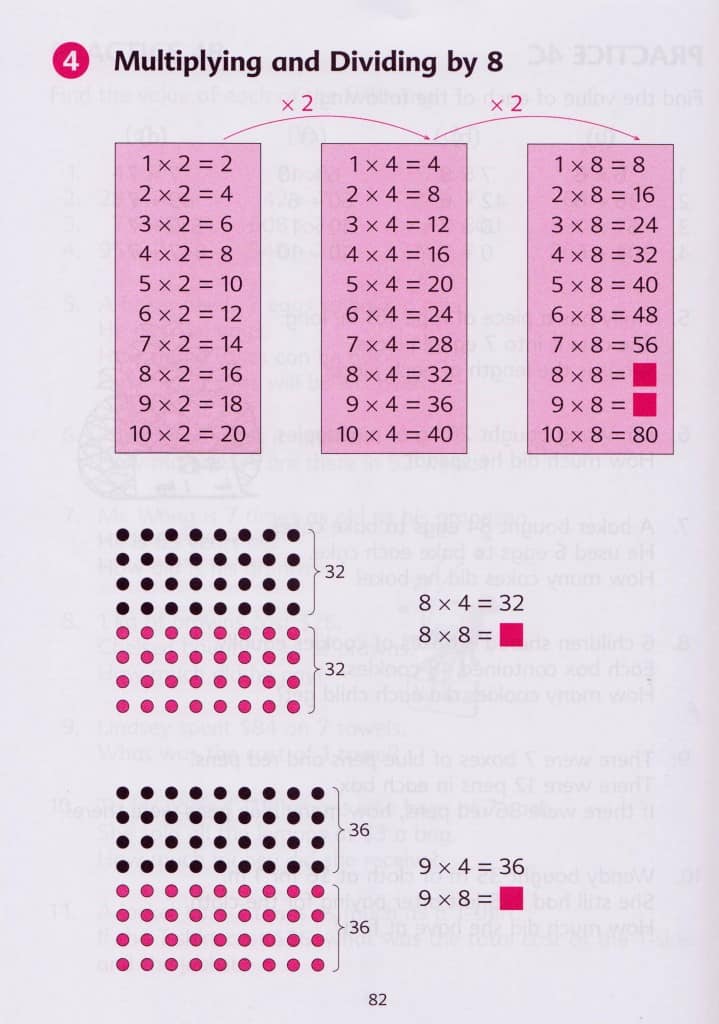
 This week’s problem comes from New Syllabus Mathematics Strategies Primary 3 by Yee Fook Shiong, published in 2007 by Educational Publishing House Pte Ltd.
This week’s problem comes from New Syllabus Mathematics Strategies Primary 3 by Yee Fook Shiong, published in 2007 by Educational Publishing House Pte Ltd.