Beth Curran – Page 4

Number Talks in the Singapore Math Classroom (Part 1)

November 21, 2016 by Beth Curran 4 Comments

Mental math plays a huge role in the Singapore Math curriculum. By developing mental math strategies in your students, you are equipping them with strong number sense, a critical skill and goal for our students to reach by the end of middle school.

You can practice mental math in your classrooms with a Number Talk; a term coined by Sherry Parrish in her popular book, Number Talks: Helping Children Build Mental Math and Computation Strategies.

Establishing Rules and Roles for a Number Talk

For Number Talks to be successful, you have to establish some rules for respectful listening and productive criticism. All students need to feel safe to participate without feeling ridiculed.

Enlisting student help when generating rules allows students to take ownership of them and creates a classroom where the rules are more likely to be followed.

To generate rules for Number Talks you might ask:

What does it look and sound like when someone is being a good listener?

It’s equally important to teach students how to respond to each other in a respectful manner. In a recent post on Edutopia, Oracy in the Classroom, types of talk were artfully organized into 6 discussion roles.

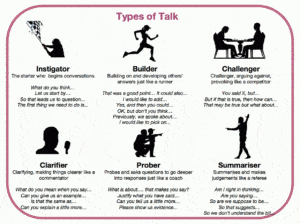
During a Number Talk, students become the Builders, Challengers and Clarifiers, while the teacher plays the roles of the Instigator, Prober, and Summariser as he or she guides the discussion as the facilitator of the Number Talk.

What should we talk about?

In Kindergarten, Number Talks can focus on subitizing and connecting the pictorial to the abstract.

Thoughtful problems are used in grades 1 through 5, designed specifically to practice mental math strategies that have been introduced in class.

In Kindergarten, show an image like this and ask, “How many dots do you see?”

In first grade, show an image like this.

Or a problem like this to practice addition strategies.

18 + 5 =

In second grade, start with a problem like this to practice addition strategies.

57 + 14 =

Or this, to practice adding a number close to 100.

97 + 33

Or this, to practice subtraction strategies.

43 – 28 =

In third grade, start with this to practice mental math with multiplication.

14 x 3 =

Or this to practice mental division.

42 ÷ 3 =

In fourth grade, start with problems like these to build on strategies learned in the previous grades.

499 + 137 =

138 – 56 =

In fifth grade, start with problems like these to continue to build on strategies learned in the previous grades.

1388 + 2983 =

29 x 7 =

135 ÷ 5 =

Give some of these a try and check back soon for the next installment of Number Talks in the Singapore Math Classroom.

Look for Part 2 next Monday!

Filed Under: Math Resources, Singapore Math Problems, Tales from the classroom, Training

Journaling in the Singapore Math Classroom

October 13, 2016 by Beth Curran 1 Comment

Communicating mathematically is a critical skill and goal for all of our students to reach by the end of middle school. In fact, Common Core Standards for Mathematical Practices, MP3, states that students will, “Construct viable arguments and critique the reasoning of other.”

Singapore’s Ministry of Education would tell you that there’s nothing Singaporean about Singapore math. When developing their highly successful math curriculum, they took theory and ideas from mathematicians and educational theorists around the world and put them into action.

What should a math journal look like?

I have attended many workshops and make-and-take sessions on planning and preparing for student math journals. Many have focused on setting up the student journal with a contents page and tabs to divide the journal into “notes,” “vocabulary” and “practice problem” sections. While this will create a journal that looks really nice, what I have found to be most effective (and one that I actually use in the classroom) is taking a simple composition or spiral bound notebook and beginning on the first page. Students make their first journal entry of the school year on page one and continue with entries on subsequent pages. Less is more!

Here’s what a journal entry page might look like:

The journal entry number just grows as the year progresses. We might come up with the title as a class, or students can create their own. The problem in the problem box can be copied by students or printed out for students to paste in their journals.

What should students put into journals?

There are four basic types of journal entries; investigative, descriptive, evaluative and creative.

Investigative: Students explore a new concept, solve a problem and make connections to prior learning.

Example: Three friends share a sleeve of cookies. Each sleeve holds 32 cookies. If each friend eats ¼ of the sleeve, how many cookies do they eat altogether?

Descriptive: Students describe or explain a concept or mathematical vocabulary.

Example: Use pictures, numbers and/or words to explain a polygon.

Evaluative: Students argue for or against a strategy or solution to explain why they think an answer is right or wrong, explain their choice of strategies or justify the most efficient strategy.

Example: Which of the strategies discussed in class today would you use to solve 245 – 97? Why?

Creative: Students write their own word problem or create their own number puzzle.

Example: The answer is 465 lbs. What’s the question?

Here’s a sample student journal page (click on image to enlarge):

When should I ask students to make journal entries?

Journaling can be a very effective tool to develop communication skills in your students. Depending on the type of entry, you could incorporate journaling into many parts of your math day. Open a class with an investigative entry to engage students. Consolidate learning and reflect on thinking with a mid-lesson descriptive or evaluative entry. Enrich students with a creative entry for early finishers of independent practice.

The benefit of journaling for the teacher is it provides a concrete formative assessment. By evaluating student responses, you can determine their readiness to handle a new task and check for understanding of concepts. Student journals also provide a great launching point for discussion at parent-teacher conferences.

_____________

Check out a resource from a previous post: Singapore Math and Math Journal Writing

Filed Under: Math Resources, Tales from the classroom Tagged With: math journals, Singapore Math curriculum, tales from the classroom

“Summer Math” Suggestions to Boost Student Understanding

June 23, 2016 by Beth Curran 4 Comments

School is out and summer is calling, but for many teachers and administrators, summer is a time to take stock and plan and budget for next year.

As a teacher, this is a glorious time of year, but also one of worry. I worry about my students. I worry about those who needed extra support throughout the year understanding and retaining math concepts. How will they fare next school year? Will they regress over the summer months if they don’t do any math work?

There are three categories of students who benefit most from summer math work:

Those who have struggled all year and maybe never quite achieved mastery on those critical grade level concepts,
those who easily forget concepts, and
those whose math confidence could use a boost.

With a Singapore Math program, there aren’t many ready-made options to pick up at the local bookstore. Books that are available focus heavily on procedural understanding rather than underlying math concepts. So what’s a teacher to do?

Aside from recommending tutoring, I have found a couple of options that seem to meet my needs as a teacher and the needs of my students.

Workbook Work

For those looking for a paper and pencil option, I recommend the Extra Practice books from Singapore Math’s Primary Mathematics series. Students should work at the grade level just completed (a rising 3rd-grade student should do summer work in the 2^nd grade Extra Practice book).

The Extra Practice books offer parents and/or tutors “Friendly Notes” at the beginning of each unit that explain how to re-teach concepts in a way that is familiar to the student. The notes are followed by practice pages that give parents sample problems appropriate for practicing the concepts and the student an option of working through problems independently. Best of all, they include an answer key in the back so parents can check work and students can re-work problems, if necessary.

These books are written to cover a year’s worth of concepts; I am by no means suggesting that a child complete the entire book over the summer. Teachers recommending this book will need to tailor the tasks to meet each student’s needs. This can be as simple as highlighting the contents page to include units or pages that you would like the student to complete over the summer keeping those critical concepts in mind.

Another option for summer work can be found in online programs. I have come across three online options for concept practice; Primary Math Digital, it’s twin Math Buddies and a program new to the US market, Matholia.

Online Options

Primary Math Digital (Free 15-day trial) and Math Buddies (Also a free trial) are backed by Singapore Math’s Primary Mathematics and Math in Focus series. Both offer students video tutorials that can be viewed by the student (and parent) an unlimited number of times. These videos are scaffolded to follow the pictorial and abstract progression of learning.

Teachers can assign videos, practice and assessment tasks f $Math Buddies$ or students to complete over the summer at their own pace. The practice pages can be a little challenging to navigate, but with some initial guidance, students should be able to complete the tasks independently.

Both programs require the school to purchase annual student and/or teacher accounts to gain access to the library of lessons. There are Homeschool accounts available. Expect a price tag of around $30 per student depending on the number of accounts purchased.

Another, more affordable option new to the US market is Matholia. Matholia was developed by two teachers from Singapore and has been used by teachers and students in Singapore as well as several other countries. This program also includes a library of video tutorials, practice and assessment tasks as well as fact fluency tasks and games.

The videos are easy to understand and are also strategically scaffolded for student understanding. The practice and assessment tasks are intuitive and easy for students to navigate. As with the other programs, teachers can assign tasks for students to complete over the summer.

Matholia also requires the school to purchase annual student accounts (teacher accounts are free) but is much more affordable at just $8 per student.

Don’t forget the concrete…

I can’t go without saying that any of these options will give students practice, but struggling students need more than just extra practice working through math problems. They need more time in the concrete phase of learning using manipulatives; base-ten blocks, place value chips, model building with connecting cubes or paper strips, fraction strips or circles, etc. So, please, consider not only sending these students home with books and login IDs but also with a bag of manipulatives for hands-on learning and practice.

Now…back to dreams of lazy mornings and time to relax and recharge. Have a great summer and rest assured that your students will be prepared for the next grade with a little summer math work.

Filed Under: Math Resources, Tales from the classroom Tagged With: materials, Singapore Math resources, tales from the classroom

How can I fit it all in?

May 19, 2016 by Beth Curran Leave a Comment

In 2015, Beth wrote about the Primary Mathematics adoption process at St. Anne’s-Belfield School. Here’s an update on the school’s progress.

As the year winds down and I look back at all that my students have learned this past year, I still feel panicked at what’s left to be covered. This is the end of a 3-year adoption cycle of Primary Mathematics and while I’ve been able to cover more curriculum than in the previous 2 years, I am still left wondering, “How can I fit it all in?”

In the fall of 2013, we adopted the Primary Mathematics curriculum in Kindergarten through sixth grades. We knew this would come with its challenges but felt strongly that if we were going to offer our students the “world’s best mathematics curriculum,” then we needed to offer it to all, not just those who made the K, 1, 2 cut.

With this plan, we knew there would be time spent filling in holes in our first year, teaching skills and concepts that the students were missing, and building a solid foundation in number sense and place value. We accepted the fact that we would not cover all of the curriculum that first year, and worked with Cassy Turner to develop a sequence for each grade level that included teaching critical lessons from prior grade levels.

Year 2

In the second year, teachers were feeling a sense of relief. We’d made it through that challenging first year. We experienced the curriculum from start to finish, well – at least our version of it, and we felt confident. We weren’t faced with the need to back-teach (as much). Our students entered the year having learned and retained a deeper understanding of those critical math concepts.

With Cassy’s advice, we created a new plan for our second year. We knew the lessons that had been skipped the previous year and teachers worked together to map out a Kindergarten through sixth-grade sequence that allowed us to get further through the content, and more importantly, accounted for previously omitted lessons. If we didn’t teach a lesson on geometry to our third graders our first year, we made sure those students would get those lessons in fourth grade our second year.

The year ended, and our standardized test scores showed slight increases in problem solving and algebra readiness, both areas of statistical concern with our previous curriculum.

Year 3

Entering year three, we felt confident in our abilities to deliver lessons. Along with our students, our staff had developed a deeper, conceptual understanding of math. We were able to effortlessly explain new concepts, differentiate on the fly, and anticipate misconceptions. We incorporated anchor tasks, journaling and finally had a grasp on how to effectively use all of the materials.

We entered the year with the goal of teaching the entire curriculum. Halfway through the year we were teaching material nearly a month ahead of our previous two years and felt really good about it. Then came…

rehearsals for performances
grandparents’ day presentations
spring field trips…
field day…
and all sorts of other school commitments.

By April, we had just about lost the scheduling lead that we had enjoyed in December.

So, here I find myself once again faced with the task of choosing one lesson over another and prioritizing the importance of skills and concepts that I may or may not have the time to teach. Fortunately, the list to choose from is smaller than in the years before. I’ve been able to cover almost all of the material, nearly reaching the goal.

I consider myself lucky to have been in a situation of specializing in math in the lower grades over the past 3 years. I have been able to experience the strengths of the sequence, which in my mind, is one of the pillars of success of a Singapore Math curriculum. Going forward, I know it will be easier to thoughtfully prioritize content to eliminate the risk of creating gaps or holes in student learning that could potentially weaken their foundation.

As I leave the school, I look forward to bringing this wealth of knowledge that I gained over the past 3 years to Math Champions and look forward to assisting other schools that are facing the question of, “How can I fit it all in?”

Filed Under: Implementing Singapore Math, Math Resources, Tales from the classroom, Training Tagged With: Singapore Math training, tales from the classroom

Anchor Tasks Demystified

February 15, 2016 by Beth Curran 5 Comments

With performance-based standards and 21st-century skill sets teachers are asked to teach mathematics with an emphasis on problems solving and inquiry learning, but how? The answer is simple, with anchor tasks, of course, BUT HOW?

I have attended several seminars and sessions that have done a great job of explaining what an anchor task is and how using anchor tasks can transform my instruction while meeting the needs of all learners. Few, however, have explained how to implement them into my daily lessons. I have been told anchor tasks are right there in the materials, but I have yet to come across a section labeled, “Anchor Task.”

In a recent seminar, hosted by Dr. Yeap Ban Har, I finally got the explanation I had been searching for… I had been looking in the wrong place! Anchor tasks are not found in the Primary Mathematics Teacher’s Guides, but rather in the textbooks.

Dr. Yeap described the evolution of the term on his Facebook page:

Basically, an Anchor Task is the concrete component of any lesson!

How do I find an Anchor Task?

In Primary Mathematics 4A, Lesson 3.6c (Standards Edition) students will learn to interpret the fraction of a set as a whole number times a fraction. The Teacher’s Guide leads teachers through an effective lesson where the teacher demonstrates how to find 1/3 of 24 using a couple of different methods.

I’ve included links to this same lesson in:

Primary Mathematics U.S. Edition: Teacher’s Guide | Textbook
Primary Mathematics Common Core Edition
Math in Focus – current edition: Part 1 | Part 2

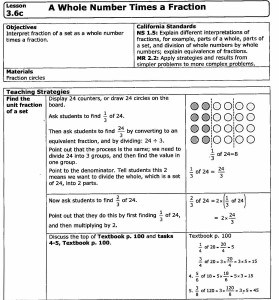
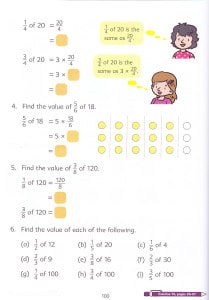To approach this lesson with more of an emphasis on inquiry learning, look to the textbook.

To create an anchor task, I took the example at the top of the page, find 1/4 of 20, and rewrote it as a word problem. Students worked in partner groups to solve the following: There are 20 M&Ms in a bag. Three friends each eat 1/4 of the bag of M&Ms. How many M&Ms did they eat altogether? Students were asked to find multiple ways of solving the problem and were given 20 chips to use if needed. Because our school has several Math Teachers that teach multiple grades, we devised a lesson planning document. (<-Click for a copy if you’d like to use it to plan your lessons)

As students worked, I circulated around the room and quickly determined which students had mastered how to find 1/4 of 20, which students still needed support with this concept and which students were able to apply that concept to find 3/4 of 20. Were they in the concrete, representational or abstract phase?

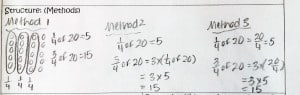After about five minutes, I gathered the students to share their methods of solving the problem. This is where my direct instruction came in. As students shared their strategies, I organized their independent learning into three methods.

I anticipated their strategies in my planning document and during my direct instruction I was sure to include any methods not discovered by my students on their own.

Students were then given the task of applying their newly discovered knowledge to solve the problems from the textbook, with my support, if needed.
The lesson ended with a journal prompt that was closely related to the concept learned.

A well-designed anchor task will engage students in the concrete and representational phases of learning a new concept.

Students will make connections with prior knowledge, reason and think logically to apply what they know to solve a problem with a partner or small group. All students will be given time to work in the concrete phase to develop and hone their conceptual understanding. As students are ready, they will naturally explore the representational or abstract phases of learning and discover strategies, or methods, for solving the given problem. Sharing methods also allows students to communicate mathematically to explain and defend their thinking and consolidate their learning.

Filed Under: Math Resources, Tales from the classroom Tagged With: Anchor Tasks, Singapore Math curriculum