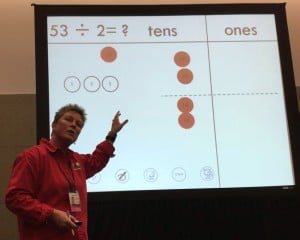
I’m thrilled to announce that I’ll be speaking at not just one, but two different international conferences at Oxford University in England this June. And I am deeply honored by the invitation to present a Keynote address at one. Here are the details:
researchED Maths and Science Conference
Saturday, June 11th, 2016 — Oxford’s Mathematical Institute
reseachED are holding an inaugural conference in Oxford aimed at primary and secondary maths and science teachers. I’ll be on a panel with Sue Lowndes and Dr. Fong Ho Kheong that will explore the implementation of the Singapore approach in non-Singaporean countries; what do we know, what we have learned and what should we do going forwards. I’ll be the representing U.S. contingent.
International Forum: Implications for UK practice in using the Singapore approach to teaching and learning in mathematics
Monday and Tuesday, June 13 – 14, 2016 — St. Anne’s College, Oxford
I will be speaking at two sessions at this conference. On Day 2, I’ll present a keynote entitled: You’ve adopted the Singapore approach to teaching mathematics – now what? Singapore maths curricula have a proven track record for meeting standards in mathematics. They can, however, be seen as a departure from prior curricula that teachers have used. This session will explore what teachers and senior leaders should consider throughout the implementation process.
I’ll also head up a workshop session, Filling in Knowledge Gaps: Critical lessons across the year groups. Upper KS2 students (that’s ages 8 to 11 to Americans) frequently lack the foundations required in order to successfully follow the Singapore curriculum. In this ‘straight from the classroom’ session, we’ll discuss the critical lessons and concepts students must master before jumping into their year-level content.
I understand that registration is brisk at these sessions. Hope to see you there!
Link to event registration pages:
- researchED Maths and Science Conference
- International Forum: Raising the bar! Implications for UK practice in using the Singapore approach to raise standards of teaching and learning in primary mathematics


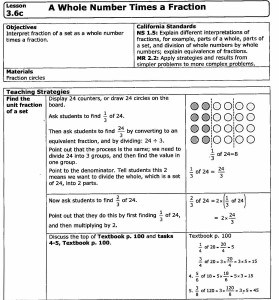
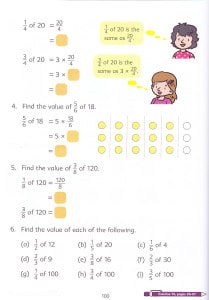 To approach this lesson with more of an emphasis on inquiry learning, look to the textbook.
To approach this lesson with more of an emphasis on inquiry learning, look to the textbook.
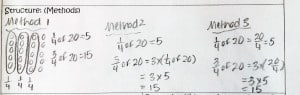 After about five minutes, I gathered the students to share their methods of solving the problem. This is where my direct instruction came in. As students shared their strategies, I organized their independent learning into three methods.
After about five minutes, I gathered the students to share their methods of solving the problem. This is where my direct instruction came in. As students shared their strategies, I organized their independent learning into three methods. Students were then given the task of applying their newly discovered knowledge to solve the problems from the textbook, with my support, if needed.
Students were then given the task of applying their newly discovered knowledge to solve the problems from the textbook, with my support, if needed.