Over the summer, we thought it would be fun to run some of the most popular posts from the past. Journaling in math class has become quite popular. We’d love to hear your successes or challenges with math journals!
Journaling in the Singapore Math Classroom
Originally published 10/13/2016
Communicating mathematically is a critical skill and goal for all of our students to reach by the end of middle school. In fact, Common Core Standards for Mathematical Practices, MP3, states that students will, “Construct viable arguments and critique the reasoning of other.”
Singapore’s Ministry of Education would tell you that there’s nothing Singaporean about Singapore math. When developing their highly successful math curriculum, they took theory and ideas from mathematicians and educational theorists around the world and put them into action.
What should a math journal look like?
I have attended many workshops and make-and-take sessions on planning and preparing for student math journals. Many have focused on setting up the student journal with a contents page and tabs to divide the journal into “notes,” “vocabulary” and “practice problem” sections. While this will create a journal that looks really nice, what I have found to be most effective (and one that I actually use in the classroom) is taking a simple composition or spiral bound notebook and beginning on the first page. Students make their first journal entry of the school year on page one and continue with entries on subsequent pages. Less is more!
Here’s what a journal entry page might look like:
The journal entry number just grows as the year progresses. We might come up with the title as a class, or students can create their own. The problem in the problem box can be copied by students or printed out for students to paste in their journals.
What should students put into journals?
There are four basic types of journal entries; investigative, descriptive, evaluative and creative.
Investigative: Students explore a new concept, solve a problem and make connections to prior learning.
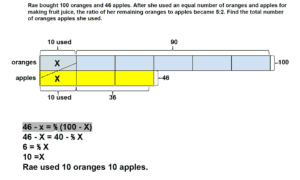
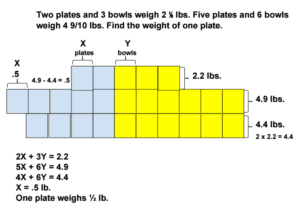
- Example: Three friends share a sleeve of cookies. Each sleeve holds 32 cookies. If each friend eats ¼ of the sleeve, how many cookies do they eat altogether?
Descriptive: Students describe or explain a concept or mathematical vocabulary.
- Example: Use pictures, numbers and/or words to explain a polygon.
Evaluative: Students argue for or against a strategy or solution to explain why they think an answer is right or wrong, explain their choice of strategies or justify the most efficient strategy.
- Example: Which of the strategies discussed in class today would you use to solve 245 – 97? Why?
Creative: Students write their own word problem or create their own number puzzle.
- Example: The answer is 465 lbs. What’s the question?
Here’s a sample student journal page (click on image to enlarge):
When should I ask students to make journal entries?
Journaling can be a very effective tool to develop communication skills in your students. Depending on the type of entry, you could incorporate journaling into many parts of your math day. Open a class with an investigative entry to engage students. Consolidate learning and reflect on thinking with a mid-lesson descriptive or evaluative entry. Enrich students with a creative entry for early finishers of independent practice.
The benefit of journaling for the teacher is it provides a concrete formative assessment. By evaluating student responses, you can determine their readiness to handle a new task and check for understanding of concepts. Student journals also provide a great launching point for discussion at parent-teacher conferences.
_____________
Check out a resource from a previous post: Singapore Math and Math Journal Writing



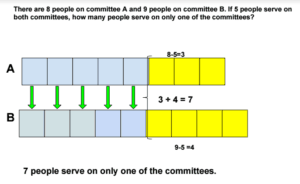


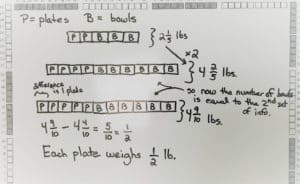







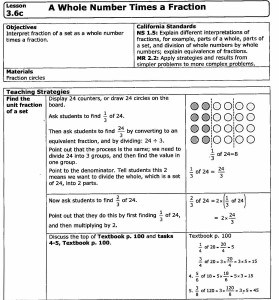
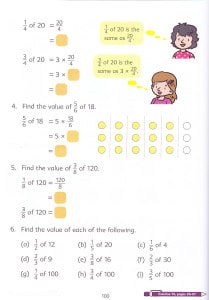 To approach this lesson with more of an emphasis on inquiry learning, look to the textbook.
To approach this lesson with more of an emphasis on inquiry learning, look to the textbook.
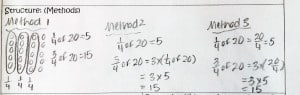 After about five minutes, I gathered the students to share their methods of solving the problem. This is where my direct instruction came in. As students shared their strategies, I organized their independent learning into three methods.
After about five minutes, I gathered the students to share their methods of solving the problem. This is where my direct instruction came in. As students shared their strategies, I organized their independent learning into three methods. Students were then given the task of applying their newly discovered knowledge to solve the problems from the textbook, with my support, if needed.
Students were then given the task of applying their newly discovered knowledge to solve the problems from the textbook, with my support, if needed.